Using Exponential Model to Describe Weight Loss

An additive logistic regression model that focuses on the interpretation of AdaBoost as a stage-wise estimation procedure for fitting an additive. Use the parameter controls in this live figure to try to fit this model to the data.

C5b2gi7ifzblxm

The simplest form of an exponential smoothing formula is given by.

. Estimates from the model were used to compare concurrent obesity regimens using 95 confidence intervals taking into account pre-determined minimal clinically important differences. Exponential regression is used to model situations in which growth begins slowly and then accelerates rapidly without bound or where decay begins rapidly and then slows down to get closer and closer to zero. In fact investigation of three individual curves from the mixed effects fractional polynomial model 1d revealed one infant boy X with a typical pattern of neonatal weight loss whose individual curve failed to capture this phenomenon Fig.

This exponential model may provide accurate estimates of maximum achievable weight loss or. This may reflect the inability of these models to describe weight loss in the first two weeks of life. On each round it makes a prediction based.

1 M DRY t M DRY 0 e-k DRY t In which M DRY t. α smoothing factor of data. 0 α 1.

For a policy of Type 1 the loss amount per year follows an exponential distribution with mean 200 and for a policy of Type 2 the loss amount per year follows a Pareto distribution with parameters alpha3 and theta200. Thus one can assume that the dry weight loss of tomato leaves in soil can be modelled by using an exponential law Jenny et al 1949. S t-1 previous smoothed statistic.

The variogram of an exponential model never reaches a sill so its range influence is uncertain. In recent years many if not most statistical and machine learning methods have been based in one way or another on the optimization of an objective or loss functionFor instance in the simplest form of linear regression given examples x 1 y 1. HEC-HMS Technical Reference Manual.

Exponential model 0996 Yes 0996 is very close tol. And the third prior days weight equals 1-094 094 2 530. Is exponential loss the only reason that leads to that adaptive algorithm concept.

The exponential model similar to the spherical model starts from the origin of the coordinates and has a linear behavior near itBut the ascending slope of the curve is less than that of the spherical model. Dydtky Multiply both sides by dt and divide both sides by y. The model could also be used to assess dose-response relationships.

The Exponential Weights Algorithm 3 33 The Exponential Weight Algorithm In plain-ish English. Loss-functions boosting ensemble. Here we propose a novel computational model the exponential weight updating EU model that addresses the limitations of existing models.

Once the best model has been found it can be used for prediction. Using the function that we have estimated using the Exponential Growth curve if we want to predict for 2 weeks after the last day of the dataset day 68 we simply put t 68 into the formula and the model predicts 3355 infections on that day. Dyyk dt Then we integrate both sides.

Given a set of experts and their predictions the Exponential Weight Algorithm2 begins with equal weights for each. Intdyyintk dt Hopefully youve learned how to anti-derive dyy and kdt. The principle has wide applicability in pharmacology physiology nutrition biochemistry and system dynamics.

Now we use the exponential model to make predictions about the number of bald eagle mating pairs. X m y m where x i ℝ n and y i ℝ one seeks to find a weight vector w. For a policy chosen at random from the entire collection of both types of policies find the probability that the annual loss will be less than.

It applies whenever a drug or nutrient is infused or ingested at a relatively constant rate and when a constant fraction. In this case 6 multiplied by 94 564. Initial dry matter weight k DRY.

2000 has a specific section Sect. 7 Loss Minimization and Generalizations of Boosting. The transient fast weight loss phase is given by.

Quadratic model 0. We use the command ExpReg on a graphing utility to fit an exponential function to a set of data points. The next squared return is simply a lambda-multiple of the prior weight.

Loss rate constant decomposition rate. Identify the best mathematical model with its corresponding R value and tell whether it is a good model. S t αx t 1 αs t-1 s t-1 α x t s t-1 Here s t smoothed statistic it is the simple weighted average of current observation x t.

Dw fast dt k 1 w fast P maxfast e k 1t E maxfast e k efft ð2Þ where w fast is the transient fast weight loss k 1 is the transient body weight gainloss rate constant P max fast is the maximum transient placebo effect E max fast is the maximum transient. Use exponential functions to model growth and decay in applied problems. So Predicted y 121 1083 t and Predicted y 1211083 t mean the same thing.

Use separation of variables to solve a simple differential equation. Differential Equations In Section 61 you learned to analyze the solutions visually of differential equations using slope fields and to approximate solutions numerically using Eulers Method. The plateau principle is a mathematical model or scientific law originally developed to explain the time course of drug action pharmacokinetics.

By using multiple model comparison methods including post-hoc model fits criterion and parameter recovery we showed that the EU model outperforms the existing models. In this equation the t-variable is an exponentSometimes you will see this written with the caret symbol. Dry matter weight at time t M DRY0.

We can solve the differential equation by first separating the variables. Tweedie as a Compound Poisson Model Claim Count N Poissonλ Claim Severity Z Gammaαθ KPW Loss Models parameters Translate into standard Tweedie parameters This is the same as predicted by well known collective risk model variance formulas 2 1 2 12 p p p p α λαθ μλαθ φ. The equation of the exponential model is Predicted eagle pairs 121 1083 t.

Quadratic nmodel 0994 No 0994 is too high an R value. Infiltration and Runoff Volume. This can be solved in the given terms to produce a general exponential growthdecay model.

In the last section we looked at linear models models that are used to describe situations in which the change in the dependent variable is a multiple m of the change in the independent variable. Weight Loss 175 120 95 63 44 33 exponential model 0996 No 0966 is too high an R value.

Pin On Health Weight Loss Muscle Gains

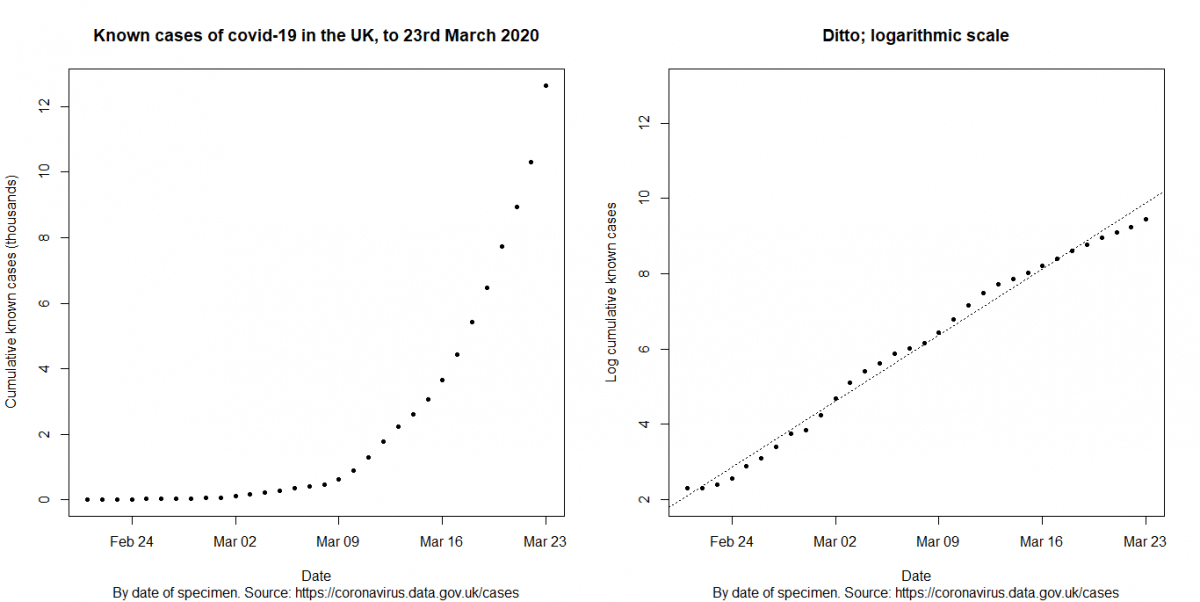
Exponential Growth What It Is Why It Matters And How To Spot It The Centre For Evidence Based Medicine

Relativity 3 Relatable Nerd Jokes Math About Me